Solving square root and other radical equations quick check embarks on an enlightening journey into the captivating world of mathematics, where we unravel the mysteries of these enigmatic expressions. This comprehensive guide empowers you with the knowledge and techniques to conquer these equations with confidence, unlocking a deeper understanding of their fundamental concepts and applications.

Delve into the intricacies of square roots and radical equations, unraveling their mathematical essence and exploring the nuances that distinguish rational and irrational numbers. Discover the power of two primary methods for solving square root equations: isolating the radical and squaring both sides.

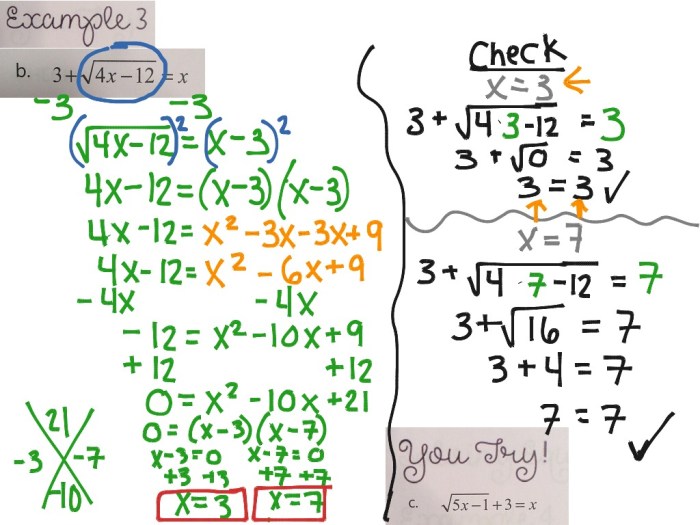
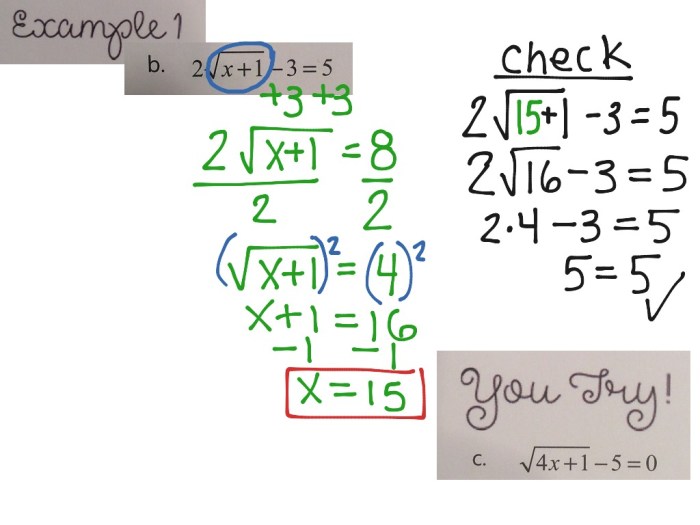
Witness the step-by-step unraveling of these techniques through illustrative examples, gaining a practical understanding of their implementation.

Solving Square Root and Other Radical Equations: Solving Square Root And Other Radical Equations Quick Check

Solving square root and radical equations is a fundamental skill in algebra. This article provides a comprehensive overview of the concepts, methods, and techniques involved in solving these equations.

Defining Square Roots and Radical Equations

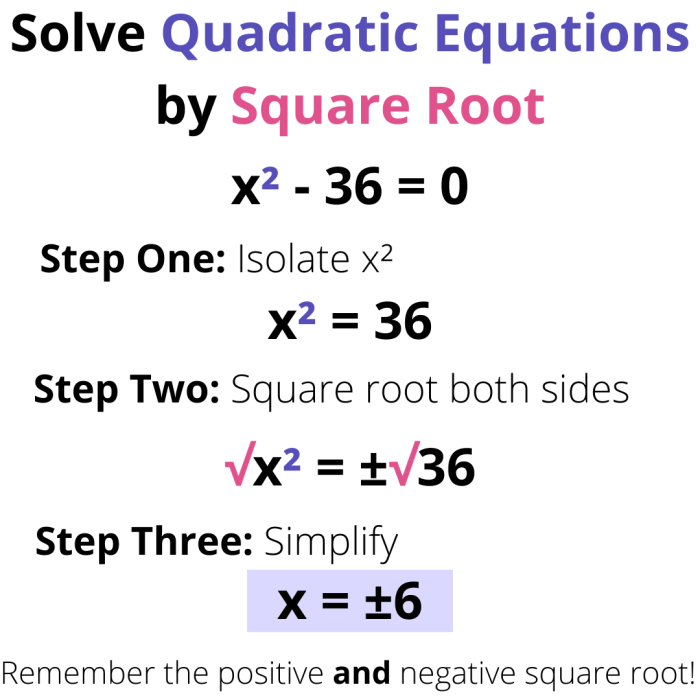
A square root is a number that, when multiplied by itself, produces the original number. It is represented by the radical symbol √. A radical equation is an equation that contains a radical expression, which is an expression that includes a radical sign.

Radical equations can be classified into two types: rational and irrational. A rational radical equation is an equation that can be simplified to an equation without any radicals. An irrational radical equation is an equation that cannot be simplified to an equation without any radicals.

Methods for Solving Square Root Equations, Solving square root and other radical equations quick check

There are two main methods for solving square root equations: isolating the radical and squaring both sides.

Isolating the Radical

1. Move all the terms that do not contain the radical to one side of the equation.
2. Square both sides of the equation to eliminate the radical.
3. Solve the resulting equation for the variable.

Squaring Both Sides

1. Square both sides of the equation.
2. Simplify the equation and solve it for the variable.

Solving Other Radical Equations

Some radical equations cannot be solved using the methods described above. These equations require rationalizing the denominator.

Rationalizing the denominator is a process of multiplying the numerator and denominator of a fraction by a suitable expression to make the denominator a rational number.

To rationalize the denominator of a radical expression, multiply the numerator and denominator by the conjugate of the denominator. The conjugate of a binomial expression is the same expression with the signs of the terms changed.

Helpful Answers

What is the difference between a rational and an irrational number?

Rational numbers can be expressed as a fraction of two integers, while irrational numbers cannot be expressed in this way and have non-terminating, non-repeating decimal expansions.

Why is it important to rationalize the denominator of a radical expression?

Rationalizing the denominator simplifies the expression and makes it easier to perform operations such as addition, subtraction, and multiplication.

Can you provide an example of a square root equation?

Sure. Here’s an example: x^2 = 9. To solve this equation, we can take the square root of both sides, resulting in x = ±3.